2021 QUARTER 01

A B C D E F G H I J K L M N O P R S T U V W
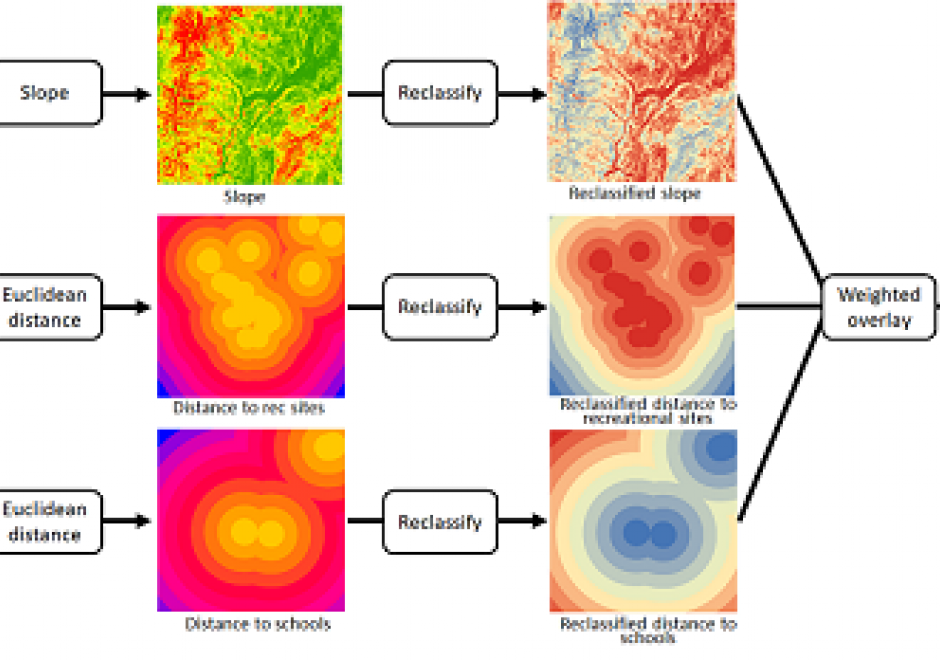
PD-33 - GDAL/OGR and Geospatial Data IO Libraries

Manipulating (e.g., reading, writing, and processing) geospatial data, the first step in geospatial analysis tasks, is a complicated step, especially given the diverse types and formats of geospatial data combined with diverse spatial reference systems. Geospatial data Input/Output (IO) libraries help facilitate this step by handling some technical details of the IO process. GDAL/OGR is the most widely-used, broadly-supported, and constantly-updated free library among existing geospatial data IO libraries. GDAL/OGR provides a single raster abstract data model and a single vector abstract data model for processing and analyzing raster and vector geospatial data, respectively, and it supports most, if not all, commonly-used geospatial data formats. GDAL/OGR can also perform both cartographic projections on large scales and coordinate transformation for most of the spatial reference systems used in practice. This entry provides an overview of GDAL/OGR, including why we need such a geospatial data IO library and how it can be applied to various formats of geospatial data to support geospatial analysis tasks. Alternative geospatial data IO libraries are also introduced briefly. Future directions of development for GDAL/OGR and other geospatial data IO libraries in the age of big data and cloud computing are discussed as an epilogue to this entry.

DM-27 - Genealogical relationships: lineage, inheritance
  • Describe ways in which a geographic entity can be created from one or more others
  • Discuss the effects of temporal scale on the modeling of genealogical structures
  • Describe the genealogy (as identity-based change or temporal relationships) of particular geographic phenomena
  • Determine whether it is important to represent the genealogy of entities for a particular application
AM-78 - Genetic Algorithms and Evolutionary Computing

Genetic algorithms (GAs) are a family of search methods that have been shown to be effective in finding optimal or near-optimal solutions to a wide range of optimization problems. A GA maintains a population of solutions to the problem being solved and uses crossover, mutation, and selection operations to iteratively modify them. As the population evolves across generations, better solutions are created and inferior ones are selectively discarded. GAs usually run for a fixed number of iterations (generations) or until further improvements do not obtain. This contribution discusses the fundamental principles of genetic algorithms and uses Python code to illustrate how GAs can be developed for both numerical and spatial optimization problems. Computational experiments are used to demonstrate the effectiveness of GAs and to illustrate some nuances in GA design.

DM-47 - Geographic coordinate system
  • Distinguish between various latitude definitions (e.g., geocentric, geodetic, astronomic latitudes)
  • Explain the angular measurements represented by latitude and longitude coordinates
  • Calculate the latitude and longitude coordinates of a given location on the map using the coordinate grid ticks in the collar of a topographic map and the appropriate interpolation formula
  • Mathematically express the relationship between Cartesian coordinates and polar coordinates
  • Calculate the uncertainty of a ground position defined by latitude and longitude coordinates specified in decimal degrees to a given number of decimal places
  • Use GIS software and base data encoded as geographic coordinates to geocode a list of address-referenced locations
  • Locate on a globe the positions represented by latitude and longitude coordinates
  • Write an algorithm that converts geographic coordinates from decimal degrees (DD) to degrees, minutes, seconds (DMS) format
FC-22 - Geometric Primitives and Algorithms

Geometric primitives are the representations used and computations performed in a GIS that concern the spatial aspects of the data, data objects described by coordinates. In vector geometry, we distinguish in zero-, one-, two-, and three-dimensional objects, better known as points, linear features, areal or planar features, and volumetric features. A GIS stores and performs computations on all of these. Often, planar features form a collective known as a (spatial) subdivision. Computations on geometric objects show up in data simplification, neighborhood analysis, spatial clustering, spatial interpolation, automated text placement, segmentation of trajectories, map matching, and many other tasks. They should be contrasted with computations on attributes or networks.

There are various kinds of vector data models for subdivisions. The classical ones are known as spaghetti and pizza models, but nowadays it is recognized that topological data models are the representation of choice. We overview these models briefly.

Computations range from simple to highly complex: deciding whether a point lies in a rectangle needs four comparisons, whereas performing map overlay on two subdivisions requires advanced knowledge of algorithm design. We introduce map overlay, Voronoi diagrams, and Delaunay triangulations and mention algorithmic approaches to compute them.

DC-30 - Georeferencing and Georectification

Georeferencing is the recording of the absolute location of a data point or data points. Georectification refers to the removal of geometric distortions between sets of data points, most often the removal of terrain, platform, and sensor induced distortions from remote sensing imagery. Georeferencing is a requisite task for all spatial data, as spatial data cannot be positioned in space or evaluated with respect to other data that are without being assigned a spatial coordinate within a defined coordinate system. Many data are implicitly georeferenced (i.e., are labeled with spatial reference information), such as points collected from a global navigation satellite system (GNSS). Data that are not labeled with spatial reference information can be georeferenced using a number of approaches, the most commonly applied of which are described in this article. The majority of approaches employ known reference locations (i.e., Ground Control Points) drawn from a reliable source (e.g., GNSS, orthophotography) to calibrate georeferencing models. Regardless of georeferencing approach, positional error is present. The accuracy of georeferencing (i.e., amount of positional error) should be quantified, typically by the root mean squared error between ground control points from a reference source and the georeferenced data product.

AM-20 - Geospatial Analysis and Model Building

Spatial modeling is an important instrument to conduct geospatial analysis to understand the world and guide decision-making. In GIS, spatial models are formal languages to express mechanisms of geographic processes and design analytical workflows to understand these processes. With the development of GIS and computer science, various types of spatial models and modeling techniques have become available, which endows the term of “spatial model” with different meanings. This entry provides an overview of common types of spatial models, modeling techniques, and related applications.

DM-71 - Geospatial Data Conflation

Spatial data conflation is the process of combining overlapping spatial datasets to produce a better dataset with higher accuracy or more information. Conflation is needed in many fields, ranging from transportation planning to the analysis of historical datasets, which require the use of multiple data sources. Geospatial data conflation becomes increasingly important with the advancement of GIS and the emergence of new sources of spatial data such as Volunteered Geographic Information.

Conceptually, conflation is a two-step process involving identifying counterpart features that correspond to the same object in reality, and merging the geometry and attributes of counterpart features. In practice, conflation can be performed either manually or with the aid of GIS with varying degrees of automation. Manual conflation is labor-intensive, time consuming and expensive. It is often adopted in practice, nonetheless, due to the lack of reliable automatic conflation methods.

A main challenge of automatic conflation lies in the automatic matching of corresponding features, due to the varying quality and different representations of map data. Many (semi-)automatic feature methods exist. They typically involve measuring the distance between each feature pair and trying to match feature pairs with smaller dissimilarity using a specially designed algorithm or model. Fully automated conflation is still an active research field.

DA-25 - Geospatial Intelligence and National Security

GIS&T exists within the national security enterprise as a multidisciplinary field that is now commonly referred to as Geospatial Intelligence (GEOINT).  U.S. GEOINT operations are principally managed by the National Geospatial-Intelligence Agency (NGA). GEOINT is one among several types of intelligence produced in support of national security, along with Human Intelligence (HUMINT), Signals Intelligence (SIGINT), Measurement and Signatures Intelligence (MASINT), and Open Source Intelligence (OSINT). Primary technical GEOINT skill areas include remote sensing, GIS, data management, and data visualization. The intelligence tradecraft is historically characterized as a process involving tasking, collection, processing, exploitation, and dissemination (TCPED), and supports decision-making for military, defense, and intelligence operations. The GEOINT enterprise utilizes every type of data collection platform, sensor, and imagery to develop intelligence reports. GEOINT products are used to support situational awareness, safety of navigation, arms control treaty monitoring, natural disaster response, and humanitarian relief operations. Geospatial analysts employed in government positions by NGA or serving in the U.S. armed forces are required to qualify in NGA’s GEOINT Professional Certification (GPC) program, and industry contractors have the option of qualifying under the United States Geospatial Intelligence Foundation (USGIF) Certified GEOINT Professional (CGP) program.

CP-05 - Geospatial Technology Transfer Opportunities, and a Case Study of the Taghreed System

The technology transfer process moves research ideas from preliminary stages in research labs and universities to industrial products and startup companies. Such transfers significantly contribute to producing new computing platforms, services, and geospatial data products based on state-of-the-art research. To put technology transfer in perspective, this entry highlights key lessons learned through the process of transferring the Taghreed System from a research and development (R&D) lab to an industrial product. Taghreed is a system that supports scalable geospatial data analysis on social media microblogs data. Taghreed is primarily motivated by the large percentage of mobile microblogs users, over 80%, which has led to greater availability of geospatial content in microblogs beyond anytime in the digital data history. Taghreed has been commercialized and is powering a startup company that provides social media analytics based on full Twitter data archive.

Pages