2021 QUARTER 02

A B C D E F G H I J K L M N O P R S T U V W
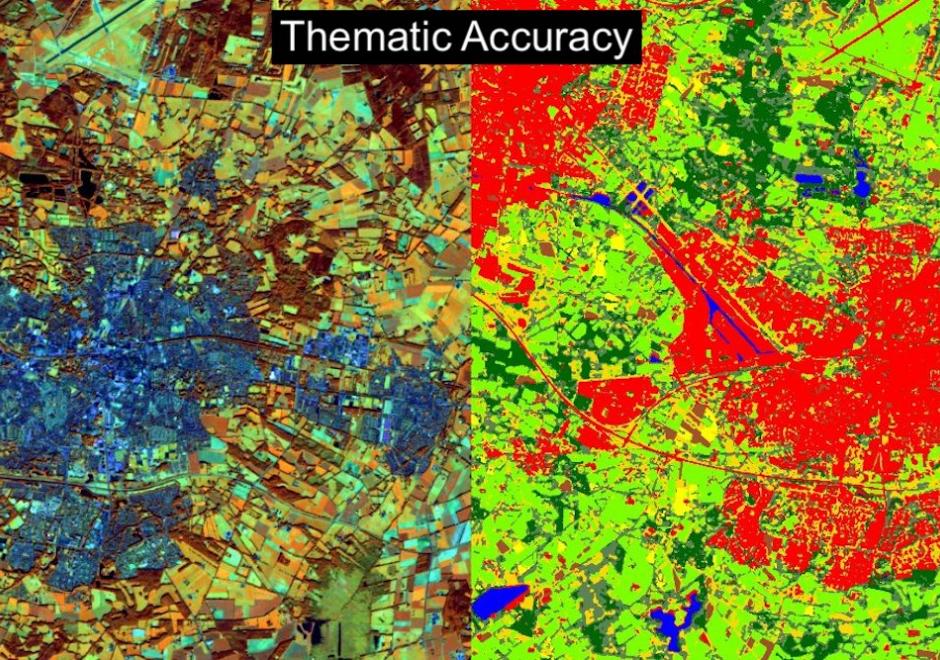
DM-07 - The Raster Data Model

The raster data model is a widely used method of storing geographic data. The model most commonly takes the form of a grid-like structure that holds values at regularly spaced intervals over the extent of the raster. Rasters are especially well suited for storing continuous data such as temperature and elevation values, but can hold discrete and categorical data such as land use as well.  The resolution of a raster is given in linear units (e.g., meters) or angular units (e.g., one arc second) and defines the extent along one side of the grid cell. High (or fine) resolution rasters have comparatively closer spacing and more grid cells than low (or coarse) resolution rasters, and require relatively more memory to store. Active research in the domain is oriented toward improving compression schemes and implementation for alternative cell shapes (such as hexagons), and better supporting multi-resolution raster storage and analysis functions.

DM-13 - The topological model
  • Define terms related to topology (e.g., adjacency, connectivity, overlap, intersect, logical consistency)
  • Describe the integrity constraints of integrated topological models (e.g., POLYVRT)
  • Discuss the historical roots of the Census Bureau’s creation of GBF/DIME as the foundation for the development of topological data structures
  • Explain why integrated topological models have lost favor in commercial GIS software
  • Evaluate the positive and negative impacts of the shift from integrated topological models
  • Discuss the role of graph theory in topological structures
  • Exemplify the concept of planar enforcement (e.g., TIN triangles)
  • Demonstrate how a topological structure can be represented in a relational database structure
  • Explain the advantages and disadvantages of topological data models
  • Illustrate a topological relation
DM-10 - The Triangulated Irregular Network (TIN) model
  • Describe how to generate a unique TIN solution using Delaunay triangulation
  • Describe the architecture of the TIN model
  • Construct a TIN manually from a set of spot elevations
  • Delineate a set of break lines that improve the accuracy of a TIN
  • Describe the conditions under which a TIN might be more practical than GRID
  • Demonstrate the use of the TIN model for different statistical surfaces (e.g., terrain elevation, population density, disease incidence) in a GIS software application
FC-27 - Thematic Accuracy Assessment

Geographic Information System (GIS) applications often involve various analytical techniques and geographic data to produce thematic maps for gaining a better understanding of geospatial situations to support spatial decisions. Accuracy assessment of a thematic map is necessary for evaluating the quality of the research results and ensuring appropriate use of the geographic data. Thematic accuracy deals with evaluating the accuracy of the attributes or labels of mapped features by comparing them to a reference that is assumed to be true. The fundamental practice presents the remote sensing approach to thematic accuracy assessment as a good guidance. For instance, the accuracy of a remote sensing image can be represented as an error matrix when the map and reference classification are conducted based on categories. This entry introduces basic concepts and techniques used in conducting thematic accuracy with an emphasis on land cover classification based on remote sensing images. The entry first introduces concepts of spatial uncertainty and spatial data quality standards and further gives an example of how spatial data quality affects thematic accuracy. Additionally, the entry illustrates the techniques that can be used to access thematic accuracy as well as using spatial autocorrelation in thematic accuracy sampling design.

AM-86 - Theory of error propagation
  • Describe stochastic error models
  • Exemplify stochastic error models used in GIScience
FC-08 - Time

Time is a fundamental concept in geography and many other disciplines. This article introduces time at three levels. At the philosophical level, the article reviews various notions on the nature of time from early mythology to modern science and reveals the dual nature of reality: external (absolute, physical) and internal (perceived, cognitive). At the analytical level, it introduces the measurement of time, the two frames of temporal reference: calendar time and clock time, and the standard time for use globally. The article continues to discuss time in GIS at the practical level. The GISystem was first created as a “static” computer-based system that stores the present status of a dynamic system. Now, GISystems can track and model the dynamics in geographical phenomena and human-environment interactions. Representations of time in dynamic GISystems adopt three perspectives: discrete time, continuous time and Minkowski’s spacetime, and three representations: ordinal, interval, and cyclical. The appropriate perspective and representation depend on the observed temporal patterns, which can be static, oscillating, chaotic, or stochastic. Recent progress in digital technology brings us opportunities and challenges to collect, manage and analyze spatio-temporal data to advance our understanding of dynamical phenomena.

DM-28 - Topological relationships
  • Define various terms used to describe topological relationships, such as disjoint, overlap, within, and intersect
  • List the possible topological relationships between entities in space (e.g., 9-intersection) and time
  • Use methods that analyze topological relationships
  • Recognize the contributions of topology (the branch of mathematics) to the study of geographic relationships
  • Describe geographic phenomena in terms of their topological relationships in space and time to other phenomena
CV-10 - Typography

The selection of appropriate type on maps, far from an arbitrary design decision, is an integral part of establishing the content and tone of the map. Typefaces have personalities, which contribute to the rhetorical message of the map. It is important to understand how to assess typefaces for their personalities, but also to understand which typefaces may be more or less legible in a labeling context. Beyond the choice of typeface, effective map labels will have a visual hierarchy and allow the user to easily associate labels to their features and feature types. The cartographer must understand and modify typographic visual variables to support both the hierarchy and label-feature associations.

Pages